WHAT?
Johannes Kepler, the assistant to Tycho Brahes in Prague, empirically recognized three legal relationships between the planets and their orbits around the sun after persistent analysis of the measurements made by his teacher:
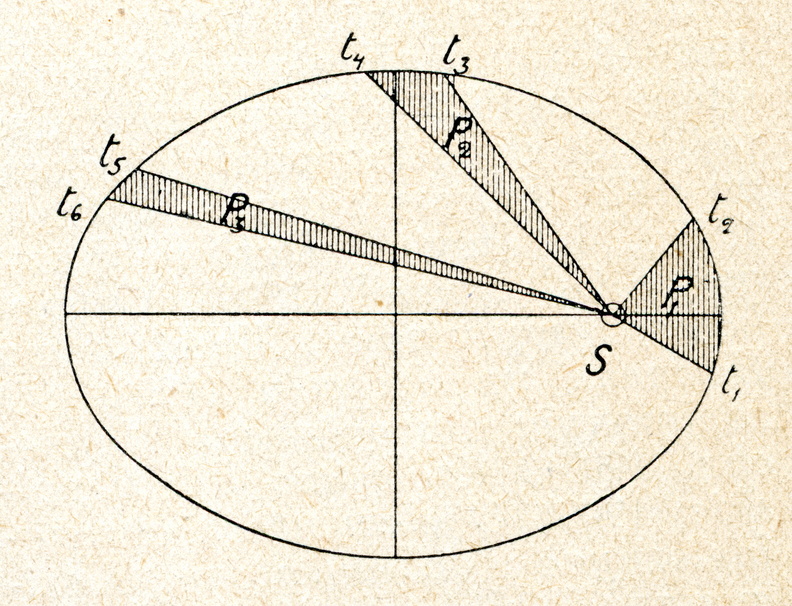
1) All planets orbit the sun in elliptical orbits. The sun is at one of the focal points of this ellipse.
2) The planets continuously change their speed during the orbit around the sun. They are faster near the sun than further away from it. The areas covered by a line between the sun and the planet are always the same for any period of time.
3) The orbital period of a planet around the sun is related to its mean distance from it: The square of the orbital period is proportional to the third power of the distance.
HOW?
Kepler found out the laws he described empirically, i.e. by observing and evaluating measurements. He could not yet give a reason for these harmonic relationships. Only Galileo Galilei and Isaac Newton came closer to a causal explanation. On the one hand, Galileo recognized that an undisturbed movement continues at a constant speed, on the other hand, Newton concluded that a change in a movement can only be done by a corresponding force.
WHERE?
Knowing the duration of a planet's orbit, you can determine its average distance from the sun and vice versa. For example, the planet Jupiter takes about 11.9 years to orbit the sun. If we choose the distance from the earth to the sun as the astronomical unit (AE), we get:
(T earth ) 2 = constant x (r earth ) 3 ,
so (1 year) 2 = constant x (1AE) 3
and (T Jupiter ) 2 = (11.9 years) 2 = constant x (r Jupiter ) 3
If you solve the first equation after the constant and insert it accordingly in the second equation, you simply get for r Jupiter = (11.9) 2/3 AE ≈ 5.2 AU. The distance from Jupiter to the sun is therefore slightly more than five times the distance from Earth.
REALLY?
The planets can deviate slightly from the ideal orbits, partly because the different planets influence each other and partly because relativistic effects play a role.